[nooonuii]

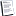
ขอปรับตัวเลขนิดหน่อยเพื่อให้โจทย์ดูง่ายขึ้น

ให้ $u=\sqrt{2}\tan\left(\frac{x}{2}\right)$ จะได้ว่า

$du=\dfrac{\sqrt{2}}{2}\sec^2\left(\frac{x}{2}\right)dx=\dfrac{2+u^2}{2\sqrt{2}}dx$

ดังนั้น $dx=\dfrac{2\sqrt{2}du}{2+u^2}$

$\sin x= 2\sin\left(\frac{x}{2}\right)\cos\left(\frac{x}{2}\right)$

$=2\tan\left(\frac{x}{2}\right)\cos^2\left(\frac{x}{2}\right)$

$=\dfrac{2\tan\left(\frac{x}{2}\right)}{\sec^2\left(\frac{x}{2}\right)}$

$=\dfrac{2\sqrt{2}u}{2+u^2}$

ดังนั้น

$\displaystyle\int\dfrac{dx}{(\sin x+\sqrt{2})^2}=\int\dfrac{1}{\left(\frac{2\sqrt{2}u}{2+u^2}+\sqrt{2}\right)^2}\dfrac{2\sqrt{2}du}{2+u^2}$

$\displaystyle =\int\dfrac{\sqrt{2}(2+u^2)}{(u^2+2u+2)^2}\,du$

ให้ $u=\tan\theta-1$ จะได้ $du=\sec^2\theta d\theta$

และ $u^2+2u+2=1+\tan^2\theta=\sec^2\theta$

ดังนั้น

$\displaystyle \int\dfrac{2+u^2}{(u^2+2u+2)^2}\,du=\int\dfrac{(\tan\theta-1)^2+2}{\sec^4\theta} \sec^2\theta d\theta$

$\displaystyle = \int(2+\cos 2\theta-\sin 2\theta)\,d\theta $

$=2\theta+\dfrac{\sin 2\theta}{2}+\dfrac{\cos 2\theta}{2}+C$

ที่เหลือแปลงกลับเอาเองนะครับ