อ้างอิง:
ข้อความเดิมเขียนโดยคุณ passer-by
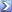
29. Compute
$$ \sum_{n=1}^{\infty} \frac{1}{n \cdot 2^n}\bigg( 1+\frac{1}{2}+\cdots \frac{1}{n} \bigg) $$
|
เมื่อคืนเบลอไปหน่อยครับ

จริงๆผมทำวิธีเดียวกับพี่passer-byเลยครับแต่ผมก็ยังติดอยู่ดี...
$\displaystyle{|x|<1\rightarrow\frac{1}{1-x}=1+x+x^2+...,-\ln(1-x)=x+\frac{x^2}{2}+\frac{x^3}{3}+...}$
$\displaystyle{\frac{-\ln(1-x)}{1-x}=x\left(1+\left(1+\frac{1}{2}\right)x+\left(1+\frac{1}{2}+\frac{1}{3}\right)x^2+...\right)}$
$\displaystyle{S=\int_{0}^{0.5}-\frac{\ln(1-x)}{x(1-x)}dx=\int_{0}^{0.5}-\left[\frac{\ln(1-x)}{1-x}+\frac{\ln(1-x)}{x}\right]dx}$
$\displaystyle{S=\frac{\ln^2 2}{2}-\int_{0}^{0.5}\frac{\ln(1-x)}{x}dx}$ ให้ $\displaystyle{I=-\int_{0}^{0.5}\frac{\ln(1-x)}{x}dx=\sum_{n=1}^{\infty}\frac{1}{n^22^n}}$
ตันครับไม่รู้อนุกรมนี้แก้ยังไง- -*
__________________
$$\int_{0}^{\frac{\pi}{2}}\frac{a\cos x-b\sin x}{a\sin x+b\cos x}dx=\ln\left(\frac{a}{b}\right)$$
BUT
$$\int_{0}^{\frac{\pi}{2}}\frac{a\cos x+b\sin x}{a\sin x+b\cos x}dx=\frac{\pi ab}{a^{2}+b^{2}}+\frac{a^{2}-b^{2}}{a^{2}+b^{2}}\ln\left(\frac{a}{b}\right)$$