อ้างอิง:
ข้อความเดิมเขียนโดยคุณ Ne[S]zA
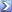
Calculate
$$ \lim_{x \to 0} \dfrac{\sqrt{1+\tan x} -\sqrt{1+\sin x}}{x^3}$$
|
ผมพึ่งฝึกเรื่องพวกนี้ช่วยดูให้หน่อยนะครับ
$\displaystyle \lim_{x \to 0} \dfrac{\sqrt{1+\tan x} -\sqrt{1+\sin x}}{x^3} = \lim_{x \to 0} \dfrac{\sin x (\dfrac{1-\cos x}{\cos x}) }{x^3 (\sqrt{1+\tan x} +\sqrt{1+\sin x})}$
$\displaystyle \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ = \lim_{x \to 0} \dfrac{\sin x}{x} \cdot \lim_{x \to 0} \dfrac{1-\cos x }{2\cos x \cdot x^2}$
$\displaystyle \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ =\lim_{x \to 0} \dfrac{1-\cos x}{2x^2}$
$\displaystyle \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ =\lim_{x \to 0} \dfrac{2-2\cos^2 \frac{x}{2}}{x^2}$
$\displaystyle \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ =\lim_{x \to 0} \dfrac{\sin^2 \frac{x}{2}}{x^2}$
$\displaystyle \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ = \dfrac{1}{4}$